Memperdalam Integral

Memperdalam Integral.

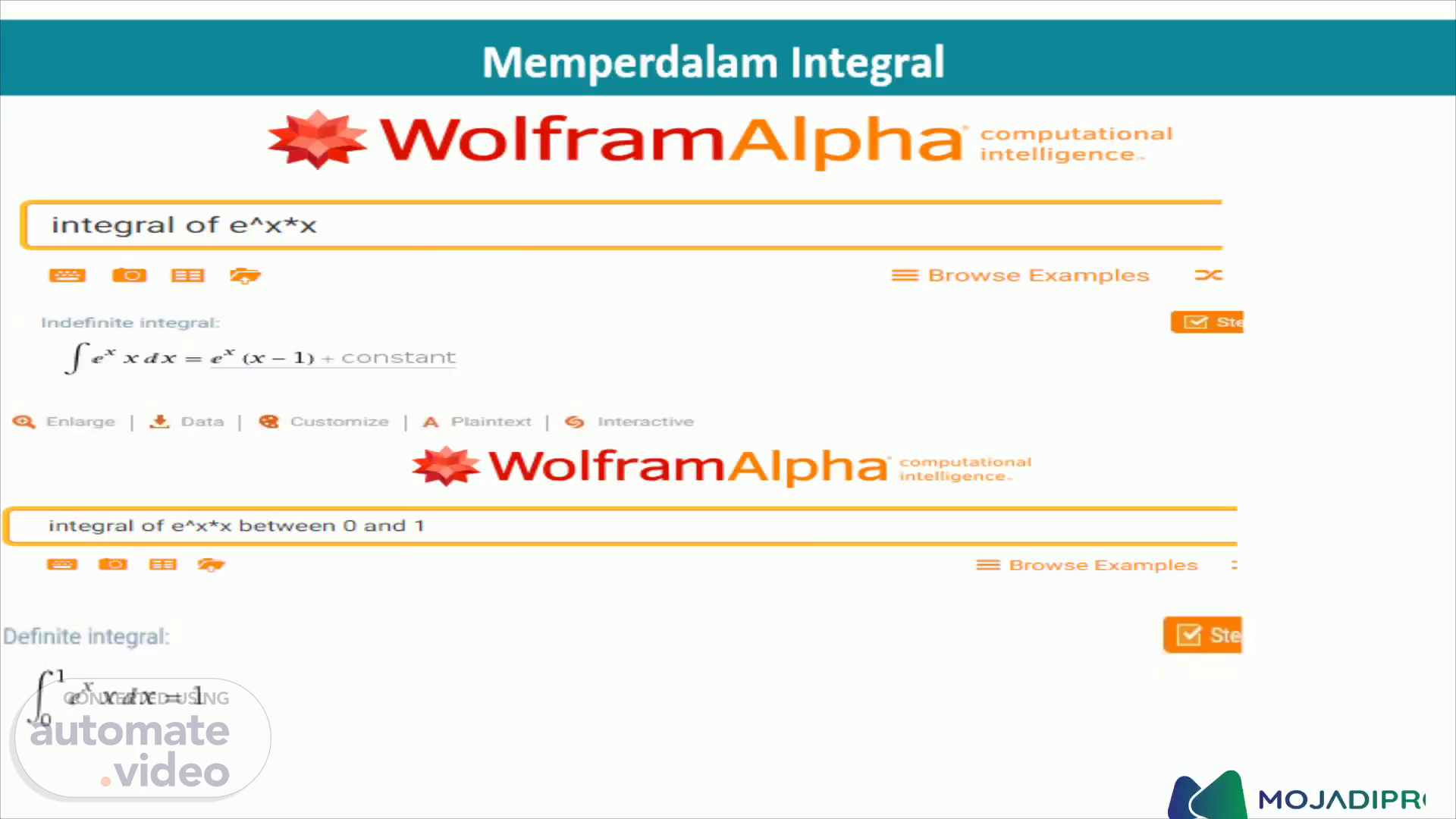
Memperdalam Integral. 1. 2. Ketik integral seperti yang kami jelaskan sebelumnyadan tekan tombol "Enter":.

Penjelasan Rumus Kompleks. 4. 5. Kita mungkin ingin menghitung nilai interval ini dalam interval tertentu dari a ke b. Dalam kasus seperti itu, kita menambahkan "antara a dan b" di akhir integral sebelum menghitung..

1. 2. Untuk variabel kontinu, ini berarti menggunakan integral dari negatif tak terhingga hingga tak terhingga. Peluang terjadinya setiap hasil diberikan oleh PDF, ? ? , so E(Y) = ׬∞ ? ? ??. −∞.

4. Karena sigma dan pi adalah bilangan konstan, kita dapat mengeluarkannya dari integral..

Distribusi Normal ? ? , ???(?). 6. 5. ??. We will substitute t in for ?−? to make the integral more manageable. To do so, we need to transform y and dy..

Distribusi Normal ? ? , ???(?). 8. 7. We solve the two simpler integrals:.

Distribusi Normal ? ? , ???(?). 9. Step 9: Using Calculus we just showed that for a variable ? which follows a Normal Distribution and has a PDF of.

Normal Distribution ? ? , ???(?). ??? ? = ? ?2 − [? ? ]2 Step 1: We already know the expected value, so we can plug in ?2 for [? ? ]2, hence: ??? ? = ? ?2 − ?2.

Normal Distribution ? ? , ???(?). Step 2: To compute the expected value for ?2, we need to go over the same process we did when calculating the expected value for ?, so let’s quickly go over the obvious simplifications..

Normal Distribution ? ? , ???(?). Step 3: We already evaluated two of the integrals when finding the expected value, so let’s just use the results and simplify..

Normal Distribution ? ? , ???(?). Step 5: The exponential tends to 0 once again, so we get the following:.

Terima Kasih Karakter Animasi - Gambar vektor gratis di Pixabay.